Lines and functions in two variables can be represented in the coordinate plane. Review the Geometry tutorial on coordinate planes if you need to brush up on that topic. In the x,y plane, the graph of an equation is the set of all pairs of points, x and y, that satisfy the equation.

The general form of a line in the coordinate plane is:

Where m is the slope of the line and b is called the y intercept. The slope can be further defined as the "rise" divided by the "run," and is a measure of how the line angles away from the horizontal. Formally, the slope is the change in the y values divided by the change in the x values: (y₂-y₁)/(x₂-x₁). A line with zero slope is parallel to the horizontal, or x axis, and thus has a slope of zero. A line with a slope of 1 rises one unit for every 1 unit that it runs. For example, consider the following line:

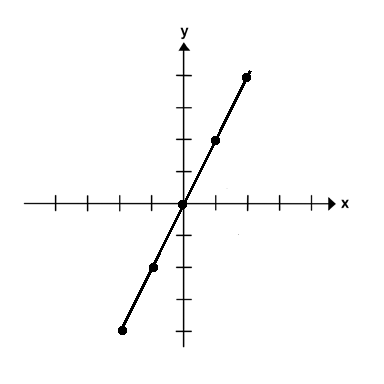
That line is composed of an infinite number of points. Several of those points, which are labeled as black dots, are (0,0), (1,2), (2,4), (-1,-2) and (-2,-4). That line has a y intercept of 0. To determine the slope visually, note that for each value that is incremented in the x direction, the y value changes by 2 points. Hence, the slope of the line is 2, and the algebra expression of the line is:

which can be simplified to:

Notice that if you were to plug in values for x and y into the equation of the line, then the resulting equation would be true.

There are two important properties of two lines that you should remember:

• Two lines are parallel if they have the same slope.
• Two lines are perpendicular if their slopes are negative reciprocals of each other. For example, the line with equation y = 3x + 4 is perpendicular to the line with equation y = -x/3.