The quadratic equation is used to solve a polynomial equation when there is a single variable which is raised to more than one power. The quadratic equation is useful when factoring and/or other simplification methods cannot work. Don't expect to use the quadratic equation often on the GRE exam, because it is one of the more advanced algebra topics that is covered on the test. For example, consider the following equation:

If you attempt to simplify this equation, you'll notice that the equation is hard to factor, and it would take a long time to find out if there are any whole number factors that can solve the equation. To solve for x, you'll need to use the quadratic equation:

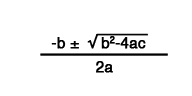
Where a is the coefficient for x2, b is the coefficient for x, and c is the coefficient without the variable.

First rewrite the equation as follows:

where we see that a = 6, b = 11, and c = -35, and solving the quadratic equation we get:

Which means that there are two possible solutions: one when the plus sign is used and the other when the negative sign is used. The solutions are (-42/12) and (20/12).

Notice that the above example required quite a few calculations, which you SHOULDN'T expect to have to do on the GRE exam. For this reason, if you find yourself using the quadratic equation, during which you are performing extensive calculations, then it's a good chance that you should not be using the quadratic equation. Remember, the questions on the GRE exam are NOT meant to be calculation intensive.